Women Mathematicians and Slow Chaos
The legacy of women mathematicians
In all the sciences, progress is a shared journey: it is the fruit of teamwork based on collaborations in the here and now as well as on the findings of researchers that have gone before.
UZH Professor Corinna Ulcigrai’s work essentially builds on the endeavors and passion of several other women mathematicians, who have inspired her (and many others) and helped her drive our understanding of slow chaos.
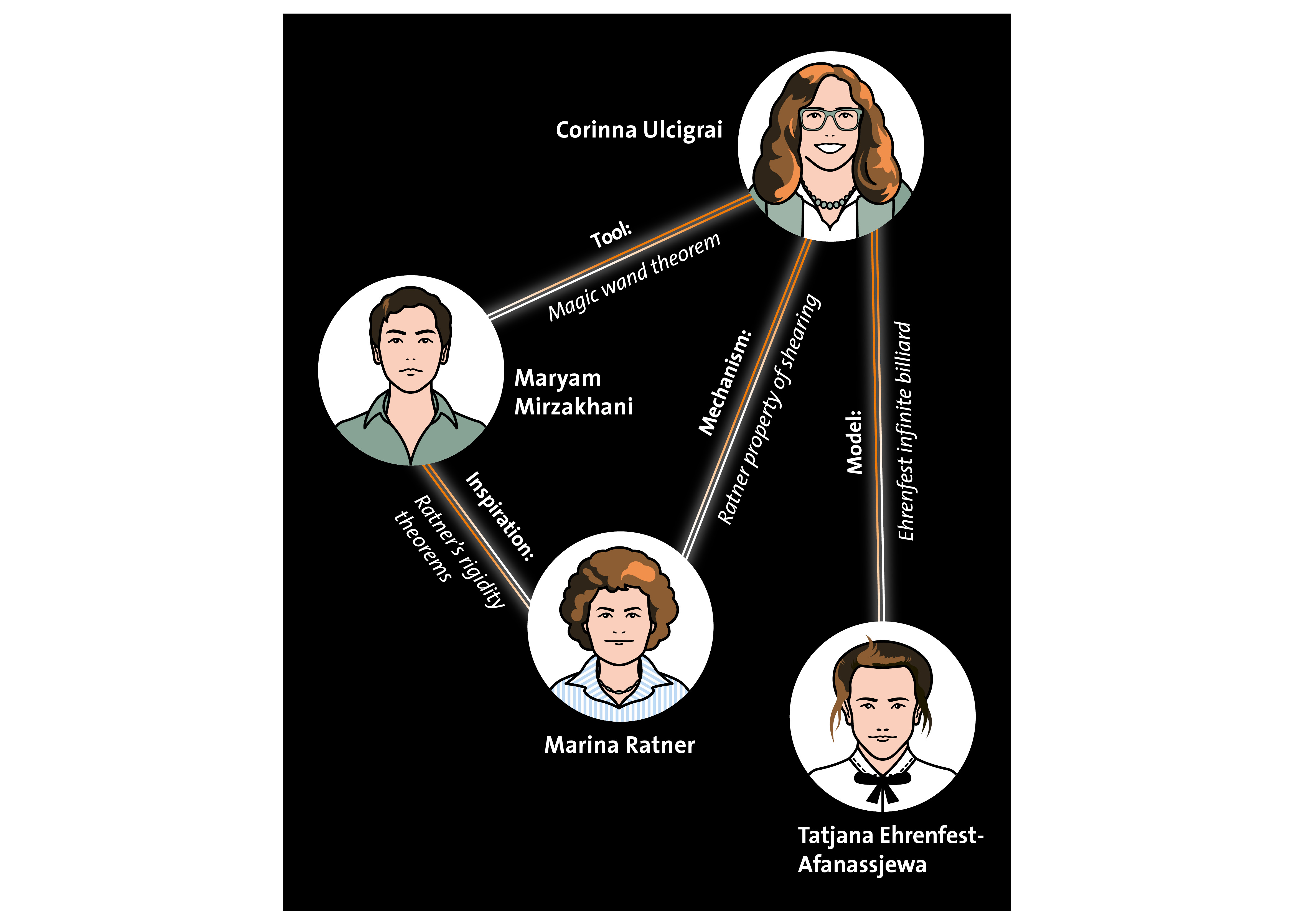
Corinna Ulcigrai's work (with co-author Fraczek) on the Ehrenfest model by Tatjana and Paul Ehrenfest uses (among other tools) a consequence of the Magic Wand theorem of Maryam Mirzakhani and co-author Alex Eskin. Another discovery (and an ongoing theme in the research of Corinna Ulcigrai and her group) is the role of a property discovered by Marina Ratner (known as the Ratner property of shearing) in the study of many slow-chaotic systems, including the Novikov model.
Maryam Mirzakhani (1977 – 2017) was an Iranian mathematician who, in 2014, became the first woman ever to be awarded the Fields Medal for, among other accomplishments, her proof known as the Magic Wand Theorem. She worked on this proof with her colleague Alex Eskin, having been inspired by the work of Marina Ratner. The Magic Wand Theorem (a tour-de-force in which geometrical properties of certain surfaces and their deformations are characterized) is one of the rare results in mathematics which uncover surprising ‘rigidity’ phenomena and is so powerful that it acts as a mathematical ‘magic wand’.
Tragically, Maryam Mirzakhani died of breast cancer at the age of 40, leaving a husband and a young daughter. In 2018, at the World Meeting for Women in Mathematics, Maryam Mirzakhani’s birthday on May 12 was chosen to be the international day on which to celebrate women in mathematics.
Find out more:
Quanta Magazine article on Maryam Mirzakhani‘s research
Movie by Zala Films featuring Maryam Mirzakhani: ‘The Secret of the Surface’
The Magic Wand Theorem: introductory paper by mathematician A. Zoric
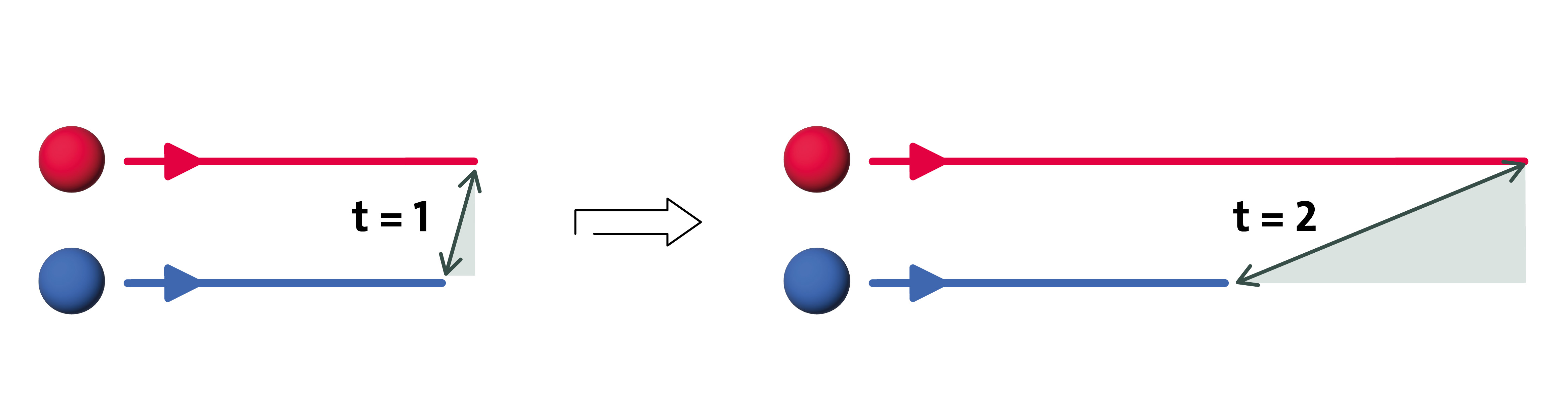
Marina Ratner (1938 – 2017), Maria Ratner (1938 – 2017), Russian-Jewish mathematician, discovered a key mechanism for slow chaos known as the “Ratner property of shearing”. The Ratner property of shearing describes the way the butterfly effect occurs in many chaotic systems: two nearby points travel along parallel trajectories, but at different speeds, such that their relative positions ‘shear’ slowly with respect to each other (see figure below).
When this type of butterfly effect occurs, for example in slowly chaotic systems of an algebraic nature, Marina Ratner was able to deduce some striking consequences. She proved many results known as ‘rigidity’ theorems, some also known as ‘Ratner’s theorems’. These have found applications in many fields of mathematics and beyond, such as number theory, mathematical physics, and networks and communications.
Marina Ratner emigrated to Israel from Russia as a single mother with a daughter, then relocated to the USA on gaining a permanent position as a professor at the University of California, Berkeley. In 1994 she became only the third woman (after Emmy Noether and Karen Uhlenberg, the first woman to win the Abel prize) to have the honor of being invited to present her work at the International Congress of Mathematicians (ICM), which took place here in Zurich.
Find out more:
Obituary in the New York Times
Women speakers at the International Congress of Mathematicians
Tatjana Ehrenfest-Afanassjewa (1876 – 1964) was a Russian-Dutch physicist and mathematician who wrote influential scientific articles on statistical mechanics. She was very interested in the didactics of mathematics and organized didactic colloquia for teachers at her home. Married to the physicist Paul Ehrenfest, she was the mathematician in the Ehrenfest research couple which, among other models, developed the infinite billiard table with rectangular obstacles in 1912. The Ehrenfest model (1912) represents slow chaos.